Volume of two cubes each with 64 cm cube,
i.e. Volume first cube = Volume of second cube = 64 cm cube
The two cubes are joined together
Since The general formula for the Volume of cube is a³ where a is the dimension of the cube
So, with the given volume, we can determine the dimension of the cube
![\begin{gathered} \text{Volume = side}^3 \\ \text{Side}^3=Volume \\ a^3=64 \\ a=\sqrt[3]{64} \\ a=4\text{ cm} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/7bozff99yz0ipfjpl5ss82tdhwvna0mxhm.png)
Thus, the side of the cube is 4cm
Since when two cube combine then the resulting figure will be :
So, the Length of the resulting figure will be equal to the twice of the side of the cube
So,
Length = 4+4 = 8 cm
Breadth= 4cm
Height = 4cm
Since we have to formulate the surface of area of the cubiod
The mathematical expression for the surface area of cubiod is :

Substitute the value and simplify
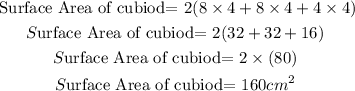
Answer : D) 160 cm²