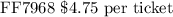The movie tickets are sold as per age groups classified into " Adult " and " Child " and " Senior ".
The movie theater compiles its sales as follows:
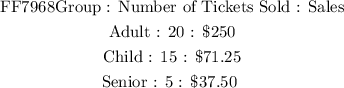
We need to determine the cost of one child ticket. To determine the rate of a child ticket to the theater using ratios as follows:
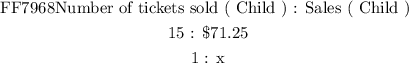
The above formulation shows that for 15 child tickets sold the theater earned $71.25. The next mathematical expression shows that if 1 child ticket was sold then (x) will the the amount earned.
We will cross multiply-( direct proportions ) the above formulation and solve for ( x ) as follows:
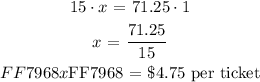
Therefore, the rate of one child ticket charged by the movie theater would be: