Let f(x) is a function and a function g(x) is given by,
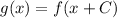
Here, if C is positive, then the graph of g(x) is the graph of f(x) shiftedC units to the left.
If C is negative, then the graph of g(x) is the graph of f(x) shifted C units to the right.
Given:
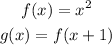
Then, g(x) can be expressed as, ,
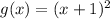
From the explanation above, g(x) is the graph of f(x) shifted 1 unit to the left.
Now, the graph of g(x) can be drawn as: