Answer:
x = -11
There aren't extran
Step-by-step explanation:
The initial equation is:
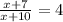
To solve the equation, we will multiply both sides by (x+10):

Then, we can apply distributive property on the right side, so:
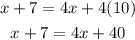
Now, we can solve the equation as:
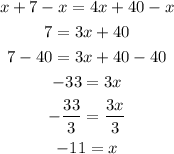
Therefore, the solution of the rational equation is x = -11
On the other hand, to identify the extraneous values, we need to replace the solutions on the initial equation, so replacing x = -11, we get:
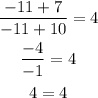
Since there isn't a problem when we replace x by -11, this solution is not an extraneous value.
So, the answer is x = -11 and there aren't extraneous values.