We know:
Profit = Revenue - Cost
When Revenue is equal to Cost, we have the break-even point.
Let's equate revenue and cost:
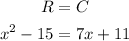
To find the value of x, we can take all terms to LHS (Left-Hand-Side) and use the quadratic formula. The process of finding x is shown below:
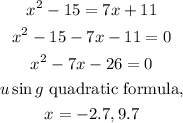
We can't have a negative value, so we disregard x = -2.7
What we have is
x = 9.7
So,
For 10 units (and above) sold, we will have a profit.
For 9 units (and below) sold, we will incur a loss.