ANSWER
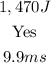
Step-by-step explanation
Parameters given:
Mass, m = 30 kg
Distance above the ground, d = 5 m
To find the amount of work that gravity does on the mass, we have to find the product of its weight and the distance of the mass above the ground:
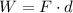
where F represents weight (since it is a force)
The weight of the mass is:

where g = acceleration due to gravity
Hence, the work done by gravity is:
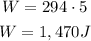
At every point during the mass's fall to the ground, it possesses both potential and kinetic energy, although in varying quantities at different points.
Hence, yes, it has energy in it.
Just before the mass hits the ground, its final kinetic energy is equal to its initial potential energy.
To find the speed of the mass, we have to first find that potential energy.
Potential energy is given by:
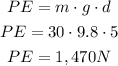
That is equivalent to its kinetic energy just before it hits the ground.
Kinetic energy is given by:

where v = speed
Now, we can solve for speed from the formula above:
![\begin{gathered} 1470=(1)/(2)\cdot30\cdot v^2 \\ (1470\cdot2)/(30)=v^2 \\ \Rightarrow v^2=98 \\ v=\sqrt[]{98} \\ v=9.9m\/s \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/x3q529ghxwnbjviiqqpfnk2ahfihkows6i.png)
That is the speed of the mass just before it hits the ground.