Question

Factor:m) yx(y+1) + 4x(y + 1) - 5(y + 1)n) 6p2 - 3p3o) 4x4 + 2x

Answer 1

`m)\left(y+1\right)\left(yx+4x-5\right)\:n)3p^2\left(2-p\right)\:o)2x\left(2x^3+1\right)`

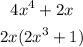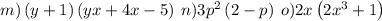
1) We can factor these expressions so that we can rewrite them as factors of a product.

m) yx(y+1) + 4x(y + 1) - 5(y + 1)

`\begin{gathered} yx\left(y+1\right)+4x\left(y+1\right)-5\left(y+1\right) \\ \left(y+1\right)\left(yx+4x-5\right) \end{gathered}`

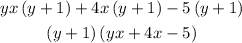
Note that there is a common factor in this expression (y+1) that repeats itself three times, so we can rewrite the whole expression using this factor.

n)6p² - 3p³

In this one, we can factor picking the GCD 3 and the least p term: p²

`\begin{gathered} n)6p²-3p³ \\ 3p^2\left(2-p\right) \end{gathered}`

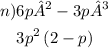
o) In this case, we can see similarities with the previous one, let's use the same process:

`\begin{gathered} 4x^4+2x \\ 2x(2x^3+1) \end{gathered}`