Let the $5.10 coffee be represented by x, and the $6.30 coffee be represented by y.
If she wishes to mix 40 pounds, this can be expressed as
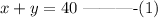
The total cost of both coffee is $222; this can be expressed as

This provides a system of equations that can be solved simultaneously:
From equation 1, we can rewrite the equation to be
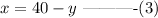
Substitute equation 3 into equation 2:
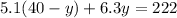
Solving, we have
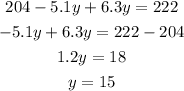
To solve for x, substitute y = 15 into equation 3:
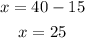
Therefore, Jamila needs 25 pounds of the $5.10 coffee and 15 pounds of the $6.30 coffee.