Question

Solve for m. Solve for the point on the graph for x=1. Solve for the equation of the tangent at x=1.

Answer 1

ANSWERS

• m = ,1

,

• The point on the graph for x = 1 is (1, ,1,)

,

• Equation of the tangent at x = 1 is ,y = x

Step-by-step explanation

First, we have to find the derivative of y,

`(dy)/(dx)=2\cdot2x^(2-1)-3\cdot1x^(1-1)+0=4x-3`

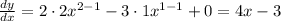
Now, to find m, we have to evaluate the derivative at x = 1,

`(dy)/(dx)\Big|_(x=1)=m=4\cdot1-3=4-3=1`

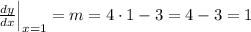
The equation of the tangent is,

`y_t=mx+b`

Where m is the slope - which we found above, and b is the y-intercept. To find b, we have to use a point on the line. We don't know what is the line, but we do know that it is tangent to the graph of y at the tangency point, whose x-coordinate is x = 1. To find the y-coordinate of the tangency point, we have to find y when x = 1,

`y=2(1)^2-3(1)+2=2-3+2=1`

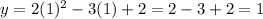
If both the graph of y and the tangent line pass through the point (1, 1), then we can use the point to find the y-intercept of the tangent line,

`1=1\cdot1+b`

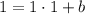
Solving for b,

`b=1-1=0`

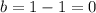
Hence, the equation of the tangent line is y = x.