Question

Finding all the zeroes of a polynomial factorand please explain1) f(x) =x^4-9x^3+32x^2-10x-52

Answer 1

Given the polynomial:

`f(x)=x^4-9x^3+32x^2-10x-52`

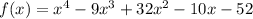
if we suppose that x = -1 is a zero of the function, when we apply sintetic division, we get:

then, our new poylinomial is:

`f(x)=(x+1)(x^3-10x^2+42x-52)`

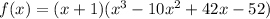
we can apply synthetic division on the second factor again if we assume that x = 2 is a zero of f(x):

so, our polynomial now looks like this:

`f(x)=(x+1)(x-2)(x^2-8x+26)`

finally, using the quadratic formula on the last factor, we get:

`\begin{gathered} x=\frac{-(-8)\pm\sqrt[]{(-8)^2-4(1)(26)}}{2(1)}=\frac{8\pm\sqrt[]{64-104}}{2} \\ =\frac{8\pm\sqrt[]{-40}}{2}=(8)/(2)\pm\frac{2\sqrt[]{10}i}{2}=4\pm\sqrt[]{10}i \end{gathered}`

therefore, the zeros of the function are:

`\begin{gathered} x=-1 \\ x=2 \\ x=4+\sqrt[]{10}i \\ x=4-\sqrt[]{10}i \end{gathered}`