Given the Complex Number:

You can identify that it is written in Rectangular Form:
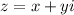
By definition, the coordinates in Rectangular Form are:
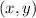
And in Polar Form:

In this case, you can identify that:
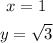
In order to convert them to Polar Form, you need to use these formulas:
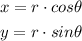
Use this formula to find "r":
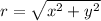
Then, this is:
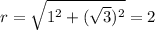
You need to find the angle. You can use this formula:
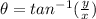
Therefore the angle is:
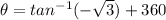
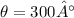
Then:
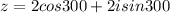
Hence, the answer is: Last option.