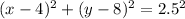Question

Please fill in the blanks of the question i sent a pic of

Answer 1

- The diameter of a circle is the longest chord of a circle.

- Thus, the coordinate of the center of the circle is the midpoint of the diameter or longest chord.

`\begin{gathered} \text{ The formula for finding the Midpoint is:} \\ M(x,y)=((x_2+x_1)/(2),(y_2+y_1)/(2)) \\ \\ (x_1,y_1)=(4,5.5) \\ (x_2,y_2)=(4,10.5) \end{gathered}`

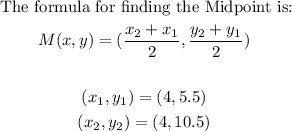
- Thus, we can solve the question as follows:

`\begin{gathered} M=(4+4)/(2),(10.5+5.5)/(2) \\ \\ M=(4,8) \end{gathered}`

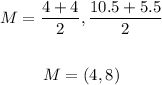
The center of the circle is (4, 8)

- The radius can be gotten by finding the distance between the coordinate of the center and any of the endpoints of the diameter.

- Thus, we have:

`\begin{gathered} D=√((x_2-x_1)^2+(y_2-y_1)^2) \\ \\ r=√((4-4)^2+(8-5.5)^2) \\ \\ r=√(2.5^2) \\ \\ r=2.5 \end{gathered}`

- The radius has a magnitude of 2.5 units

- The equation of the circle can be gotten by the formula given below:

`\begin{gathered} \text{ Equation of a circle} \\ r^2=(x-a)^2+(y-b)^2 \\ where, \\ (a,b)\text{ is the center of the circle} \\ r\text{ is the radius} \\ \\ 2.5^2=(x-4)^2+(y-8)^2 \end{gathered}`

The equation of the circle becomes:

`(x-4)^2+(y-8)^2=2.5^2`