Answer
The solution to the system of equations is:
x = -3
y = -4
The ordered pair form is: (-3, -4)
Solution
- The question tells us to find the solution to the system of equations:
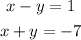
- To find the solution to the system of equations, we will apply the elimination method. This method entails adding and subtracting both equations in order to find the values of x and y.
- We can thus proceed to solve the question as follows:
Finding the value of x:
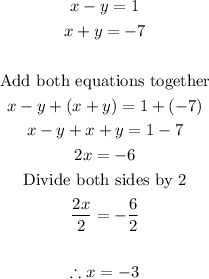
Finding the value of y:

Final Answer
The solution to the system of equations is:
x = -3
y = -4
The ordered pair form is: (-3, -4)