Question

Options for the first time:increases, remains the same, decreases Options for the second box: increases, remains the same, decreases Options for the third box: reflects over the x-axis, remains the same, reflects over the y-axis

Answer 1

The general form of a trigonometric function is:

`A\sin (B(x-C))+D`

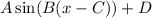
Where B is the frequency of the function.

In our problem, A=1, C=D=0.

Then, as the value of B increases, so the frequency does. The answer to the second gap is 'increases'.

On the other hand, let P be the period and f the frequency. Those two quantities are related by the formula:

`f=(1)/(P)`

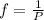
Then, if the frequency increases, the period decreases. The answer to the first gap is 'decreases'.

Finally, if B is negative we have that:

`\begin{gathered} B<0,A=-B,A>0 \\ \Rightarrow\tan (Bx)=(\sin(Bx))/(\cos(Bx))=(\sin(-Ax))/(\cos(-Ax))=-(\sin(Ax))/(\cos(Ax))=-\tan (-Bx) \end{gathered}`

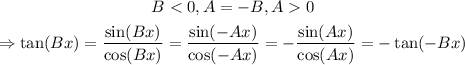
Therefore, the function is reflected over the x-axis.