Question

There were 298 tickets purchased for a major league baseball game. The general admission tickets cost $6.50 and the upper box tickets cost $10.00. The total amount of money spentwas $2234.50. How many of each kind of ticket were purchased?How many general admission tickets were purchased?How many upper box tickets were purchased?

Answer 1

Total number of ticket bought = 298

General admission ticket cost(each) = $6.50

Upper box ticket cost(each) = $10.00

Total amount spent = $2234.50

let

x = number of general admisssion tickets bought

y = number of upper box tickets bought.

Therefore,

`\begin{gathered} x+y=298 \\ 6.50x+10y=2234.50 \end{gathered}`
`\begin{gathered} x=298-y \\ \end{gathered}`

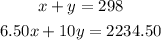
Insert the value of x in equation(ii)

`\begin{gathered} 6.50(298-y)+10y=2234.50 \\ 1937-6.50y+10y=2234.50 \\ 1937+3.50y=2234.50 \\ 3.50y=2234.50-1937 \\ y=(297.5)/(3.50) \\ y=85 \end{gathered}`
`\begin{gathered} x+85=298 \\ x=298-85 \\ x=213 \end{gathered}`

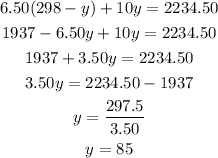
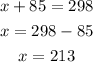
Number of general admission tickets purchase = 213

Number of upper box tickets purchased = 85