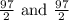Question

Among all rectangles that have a perimeter of 194 by the dimension of the one whose area is the largest write your answers as a fraction reduced to lowest terms

Answer 1

Step-by-step explanation

From the statement, we must find the dimensions of a rectangle with:

• perimeter P = 194,

,

• the largest area.

We consider a rectangle with sides x and y. We will write formulas for P (the perimeter) and A (the area). Then we will express the area in terms of one side A(x), and maximize the function.

(1) The perimeter of the rectangle is:

`P=2(x+y)\rightarrow y=(P)/(2)-x=(194)/(2)-x=97-x.`

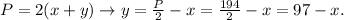
(2) The area of the rectangle is:

`A=x\cdot y=x\cdot(97-x)=-x^2+97x.`

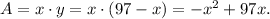
(3) To maximize the area A(x), we compute and make equal to zero its first derivative, then we solve for x:

`A^(\prime)(x)=-2x+97=0\Rightarrow2x=97\Rightarrow x=(97)/(2).`

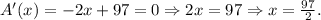
(4) From point (1), we find the length of the other side:

`y=97-x=97-(97)/(2)=(97)/(2).`Answer

The dimensions of the rectangle with a perimeter of 194 and the largest area are:

`(97)/(2)\text{ and }(97)/(2)`