Question

6. Combine the following logarithms into a single logarithm. Be sure to show all your work and explain which log properties you used for each step. Do not overly simplify.1 over 6 log subscript 4 z plus log subscript 4 open parentheses z squared minus 36 close parentheses minus 7 over 6 log subscript 4 open parentheses z minus 6 close parentheses

Answer 1

Given:

`(1)/(6)\log _4z+\log _4(z^2-36)-(7)/(6)\log _4(z-6)`

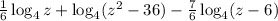
To combine these logarithms into a single logarithm, the first thing we need to do is to re-write the expression as a single logarithm with coeffient.

`(1)/(6)\log _4z+\log _4(z^2-36)-(7)/(6)\log _4(z-6)=\frac{\log _4\sqrt[6]{z}+\log _4(z^2-36)}{\log _4\sqrt[6]{(z-6)^7}}`

Next, we will use the Product Rule to simplify the numerator.

Product Rule states that:

`\log _b(MN)=\log _bM+\log _bN`

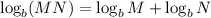
Applying it to the numerator:

`\log _4\sqrt[6]{z}+\log _4(z^2-36)=\log _4(\sqrt[6]{z}(z^2-36))`

We will now have a new expression:

`\frac{\log_4(\sqrt[6]{z}(z^2-36))}{\log_4\sqrt[6]{(z-6)^7}}`

Next, we will factor out the logarithm.

`\frac{\log_4(\sqrt[6]{z}(z^2-36))}{\log_4\sqrt[6]{(z-6)^7}}=\log _4(\frac{\sqrt[6]{z}(z^2-36)}{\sqrt[6]{(z-6)^7}})`

Therefore, the final answer would be:

`\log _4(\frac{\sqrt[6]{z}(z^2-36)}{\sqrt[6]{(z-6)^7}})`