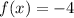Question

How to write a linear function with the values f(3)=-4 f(5)=-4

Answer 1

`f(x)=-4`

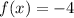
Explanation:

We have that a linear function in its slope and intercepot form is the following:

`\begin{gathered} f(x)=mx+b \\ \text{ where m is the slope and b is y-intercept} \end{gathered}`

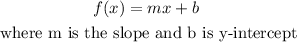
We calculate the slope as follows:

`m=\frac{f(x_2)-f(x_1)_{}}{x_2-x_1}`

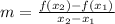
The points to use would be (3, -4) and (5, -4)

`m=(-4-(-4))/(5-3)=(-4+4)/(2)=(0)/(2)=0`

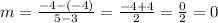
We can see that the slope is 0, which means the function would be:

`f(x)=-4`