Question

Solve: |3(x-5)+2|-3=9

Answer 1

Answer: x= 25/3

Explanation:

Answer 2

The question gives us an absolute value problem.

In order to solve the problem, we should first deal with the 3 by adding 3 to both sides so as to get the absolute value alone and apply a theorem about absolute values which will help us solve the problem.

Let us do this below:

`\begin{gathered} |3(x-5)+2|-3=9 \\ \text{Add 3 to both sides} \\ |3(x-5)+2|-3+3=9+3 \\ \therefore|3(x-5)+2|=12 \end{gathered}`

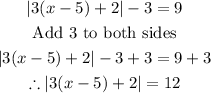
Now that we have the absolute value all alone, let us apply the theorem.

The theorem states:

`\begin{gathered} |a|=|-a|=a \\ \text{This means that any expression in the absolute value sign} \\ \text{can be either positive or negative but the answer will always} \\ be\text{ positive} \end{gathered}`

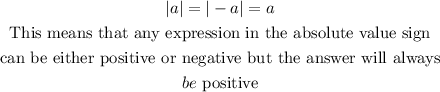
From the explanation, it is clear that there are two possible values for x because:

`\begin{gathered} \text{If 3(x-5)+2 is a negative},\text{ then we say} \\ 3(x-5)+2=-12 \\ \\ \text{if 3(x-5)+2 is positive, then we say} \\ 3(x-5)+2=12 \end{gathered}`

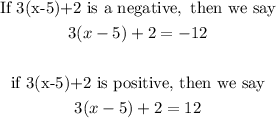
This is because if we place either 12 or -12 into the absolute value, the final answer will be 12

Therefore, let us now solve the resulting 2 equations separately and find the possible values

for x.

This is done below:

`\begin{gathered} 3(x-5)+2=12 \\ Subtract\text{ 2 to both sides} \\ 3(x-5)+2-2=12-2 \\ 3(x-5)=10 \\ \text{Expand the bracket} \\ 3x-5(3)=10 \\ 3x-15=10 \\ \text{add 15 to both sides} \\ 3x-15+15=10+15 \\ 3x=25 \\ \text{Divide both sides by 3} \\ (3x)/(3)=(25)/(3) \\ \\ \therefore x=(25)/(3) \end{gathered}`

This gives the first possible value of x. i.e. x = 25/3

The next possible value of x is gotten by making the same expression equal to -12.

`\begin{gathered} 3(x-5)+2=-12 \\ Subtract\text{ 2 from both sides} \\ 3(x-5)+2-2=-12-2 \\ 3(x-5)=-14 \\ \text{Expand the bracket} \\ 3x-5(3)=-14 \\ 3x-15=-14 \\ \text{Add 15 to both sides} \\ 3x-15+15=-14+15 \\ 3x=1 \\ \text{Divide both sides by 3} \\ (3x)/(3)=(1)/(3) \\ \\ \therefore x=(1)/(3) \end{gathered}`

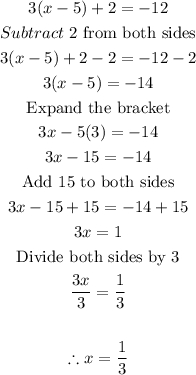
The second possible value of x is 1/3