R3 and R4 are in series, so:
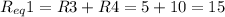
R6 and R7 are also in series, so:
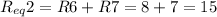
R5, Req1 and Req2 are in parallel, so:

Now, Req 3, R10 and R11 are in series, so:
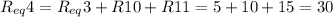
Now, we can find the total current in that segment using ohm law:
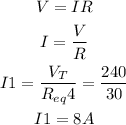
Now, let's find the voltage and the current for R3,R4, R5, R6, R7, R10 and R11
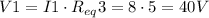
With this value, let's complete one portion of the table:
---------------
Now, R8 and R9 are in series, so:
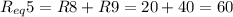
Req5 and Req4 are in parallel, so:
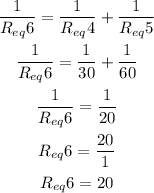
R1 and R2 are in parallel, R12 and R13 are in parallel too, so:
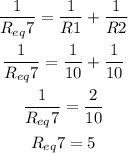

Therefore, the total resistance of the circuit since Req6, Req 7, Req8 and R14 are in series, is:
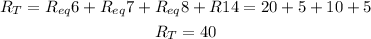
And the total current is:
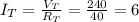
Answer: