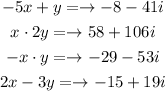
Step-by-step explanation
Let
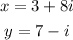
now, to multiply a imaginary number, multiply the constant
and when adding or subtracting another number, just ad/sub component to component
so
Step 1
a)-5x+y
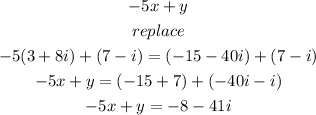
Step 2
b)x*2y

Step 3
c)-x*y
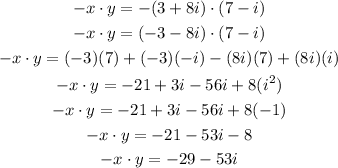
Step 4
d)2x-3y

I hope this helps you