Hello there. To solve this question, we'll have to remember some properties about logarithms.
Given the logarithmic equation:
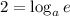
We have to determine the base a.
For this, remember that the logarithmic function is defined as
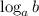
For values of a, b such that

Also, we need the following property:

Such that we have:

Take the square root on both sides of the equation
![\begin{gathered} \sqrt[]{e}=\sqrt[]{a^2} \\ |a|=\sqrt[]{e} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/p7ov7z3oej4c6040x8psqoyulyepojjz4k.png)
This gives us two solutions, according to the definition of the absolute value function:
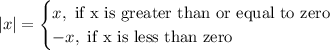
In this case, remember that a > 0, so the only solution we're interested is:
![a=\sqrt[]{e}](https://img.qammunity.org/2023/formulas/mathematics/college/en2umtfcp8p3lvyjokl2zyrdim7og0n6oz.png)
In this case, we've showed that:
![\log _{\sqrt[]{e}}e=2](https://img.qammunity.org/2023/formulas/mathematics/college/n98qise4uo64kw7rr40dipazepvfyoizjm.png)
In fact, all the other properties hold and this is the solution for the base a in this logarithmic equation.