Question

A conservation organization releases 100 animals of an endangered species into a game preserve. The organization believes that the preserve has a carrying capacity of 1000 animals and that the growth of the herd will follow the logistic curve P=1000/1+9e^-0.165tWhat is the population after 5 months

Answer 1

Given:

The growth of the herd will be modelled by the equation.

`P=(1000)/(1+9e^(-0.165t))`

Where P = Population of the herd

t = time in months

Required:

To find the population after t months.

Step-by-step explanation:

Substitute t= 5 in the given equation.

`\begin{gathered} P=(1000)/(1+9e^(-0.165(5))) \\ P=(1000)/(1+9e^(-0.825)) \end{gathered}`
`\begin{gathered} P=(1000)/(1+9(0.4382)) \\ P=(1000)/(1+9(0.4382)) \\ P=(1000)/(1+3.9438) \\ P=(1000)/(4.9438) \\ P=202.273 \\ P\approx202 \end{gathered}`

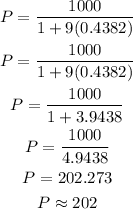
Final Answer:

The population after 5 months is 202 animals expected.