Question

I don’t understand these questions. Could you please help me? I need clearly explain.

Answer 1

We need to sketch the graph of V(t), with V in ml and t in seconds. Notice that:

`V(0)=2200`

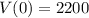
because for t = 0, her lungs start with a volume of 2200 ml.

Since the period (of a complete breath) is 4 seconds, and the function is sinusoidal, the graph is:

b. Now, a sinusoidal function has the form:

`V(t)=A\sin \lbrack(2\pi)/(T)(t-b)\rbrack+c`

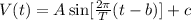
where:

• A is the amplitude (half the difference between the smallest and the largest values of V);

,

• T is the period;

,

• b is the horizontal shift (the difference between the initial value of t and the value of t for which V(t) has the mean value);

,

• c is the vertical shift (the mean value of V(t)).

So, observing the graph, we see that:

`A=(2800-2200)/(2)=300`

`T=4`

`b=1`

`c=(2200+2800)/(2)=2500`

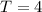
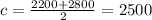
Therefore, the equation representing the volume V, in ml, after t seconds is:

`\begin{gathered} V(t)=300\sin \lbrack(2\pi)/(4)(t-1)\rbrack+2500 \\ \\ V(t)=300\sin \lbrack(\pi)/(2)(t-1)\rbrack+2500 \end{gathered}`

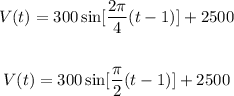
c. If Aira breathed more rapidly, the period and the horizontal shift of the function V(t) would be smaller. Thus, the graph would change more rapidly also, i.e., we would see more variations in the 20 seconds.

For example, if she breathed 2 times faster, the period would be 2 instead of 4, and the equation would be:

`\begin{gathered} V(t)=300\sin \lbrack(2\pi)/(2)(t-0.5)\rbrack+2500 \\ \\ V(t)=300\sin \lbrack\pi(t-0.5)\rbrack+2500 \end{gathered}`

And the graph would be:

And if Aire took bigger breaths, the amplitude and the vertical shift of the function V(t) would increase. For example, if her lungs could reach the volume of 3000 ml, assuming the same period of 4 seconds, the equation would be:

`V(t)=400\sin \lbrack(\pi)/(2)(t-1)\rbrack+2600`

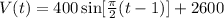
And the graph would be: