Given:
• m∠APB = 49 degrees
,
• Radius = 13 cm
Let's find the length of arc AB.
To find the length of arc AB, apply the formula:
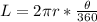
Where:
r is the radius = 13 cm
θ is the central angle = 49 degrees
Thus, we have:
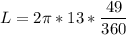
Solving further:

Therefore, the length of arc AB is 11.1 cm.
• ANSWER:
d. 11.1 cm