Given data:
* The mass of the first train car is,
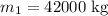
* The initial velocity of the first train car is,

* The velocity of the couple train car is,
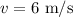
Solution:
By the law of conservation of momentum,
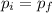
where p_i is the initial momentum of net system, and p_f is the final momentum of the net system,

where m_2 is the mass of the second train car, and u_2 is the initial velocity of the second train car,
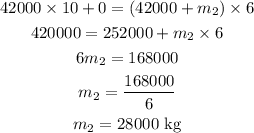
Thus, the mass of the second train car is 28000 kg.