We have the following equation to find the distance between two points A and B:
![d(A,B)=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}](https://img.qammunity.org/2023/formulas/mathematics/high-school/555cxyekk5kg869c1y2u4k9oaarw94dsj1.png)
In this case, if the points are A=(4,5), B=(10,5) and C=(4,-3), then we can find the length of the sides of the triangle using the formula for the distance between two points:
![\begin{gathered} A=(4,5) \\ B=(10,5) \\ C=(4,-3) \\ --------------------------- \\ d(A,B)=\sqrt[]{(10-4)^2+(5-5)^2}=\sqrt[]{(6)^2}=6 \\ d(A,C)=\sqrt[]{(4-4)^2+(-3-5)^2}=\sqrt[]{(-8)^2}=\sqrt[]{64}=8 \\ d(B,C)=\sqrt[]{(4-10)^2+(-3-5)^2}=\sqrt[]{(-6)^2+(-8)^2}=\sqrt[]{36+64} \\ =\sqrt[]{100}=10 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/i720dfd6z8nwj4qe77j4523no7p3np5i91.png)
then, if we add the 3 distances, we can find the perimeter of the triangle:
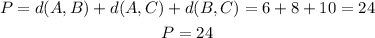
therefore, the perimeter of the triangle is 24 units