Solution:
The vertical change between two points is called the rise.
The vertical change (dy) is the difference between the y-coordinates of the points.
The horizontal change is called the run.
The horizontal change (dx) is the difference between the x-coordinates of the points.
The slope equals the rise divided by the run. The slope is also the average rate of change.
Given:
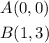
where;
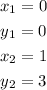
a) The vertical change (dy) is calculated as shown below;
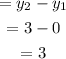
Therefore, the vertical change (rise) is 3.
b) The horizontal change (dx) is calculated as shown below;
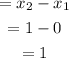
Therefore, the horizontal change (run) is 1.
c) The average rate of change (slope) is given by;
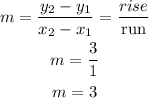
Therefore, the average rate of change (slope) is 3.