Question

Hi my name is Christopher can i get help with systems word problems?

Answer 1

Step 1: System word problem

95 packs shared among adults and children and sold for $960

Step 2: Identify each of the word problems with an equation

let x represents the number of Adult ticket

let y represents the number of a child ticket

Step 3: write out an equation for the word problem

`\begin{gathered} x+y=95--------------(1) \\ 12x+9y=960-----------(11) \\ \text{solve the simultaneous equation using substitution method considering the coefficient of y} \\ *\text{ 9 x+y=95}---------(1) \\ *1\text{ 12x+9y=960-----(11) } \end{gathered}`

Step 4: evalaute and subtract equation (11) from equation (1)

`\begin{gathered} 9x+9y=855 \\ - \\ 12x+9y=960 \\ -3x\text{ = -105} \\ \text{divide both side by -3} \\ (-3x)/(-3)\text{ = }(-105)/(-3) \\ x\text{ = 35} \\ \text{sub the value of x in equ(11)} \\ 12(35)+9y=960 \\ 420+9y=960 \\ \text{collect like terms} \\ 9y=960-420 \\ 9y=540 \\ divide\text{ both side by 9} \\ (9y)/(9)\text{ = }(540)/(9) \\ y=60 \end{gathered}`

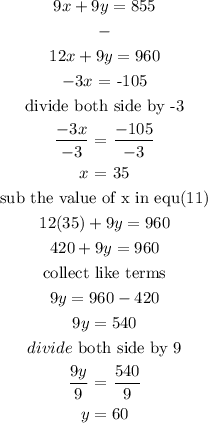
Hence the number of adult ticket = 35 while the number of child ticket = 60