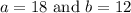1 Answer

Rivu · Answer 1 · 2023-10-10T01:00:06+0000

To find:

Find the value of and so that () is everywhere differentiable.

Solution:

The limits of f(x) at x = -1 are:

$\lim_(x\to-1^-)f(x)=\operatorname{\lim}_(x\to-1^-)(ax^2+bx+4)=a-b+4...(1)$
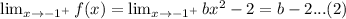
$\lim_(x\to-1^+)f(x)=\lim_(x\to-1^+)bx^2-2=b-2...(2)$

if a function is to be everywhere differentiable, then it must also be continuous everywhere. This implies that the one-sided limits must be equal.

Thus,

$\lim_(x\to-1^(-1))f(x)=\lim_(x\to-1^+)f(x)$

So, eq 1 = eq 2.

$\begin{gathered} a-b+4=b-2 \\ a-2b=-6...(3) \end{gathered}$

Next, the derivative one-sided limits must also equal at x = -1.

That is,

Thus,

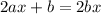
Substitute x = -1 in eq 4, then

Solve eq 3 and eq 5 for a and b.

$a=18\text{ and }b=12$

Please log in or register to add a comment.