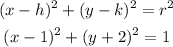Question

The center of a circle is (1,-2) and the circle is tangent to the y-axis. Find the equation of the circle in the form (x – h)2 + (y – k)2 = -2

Answer 1

From the statement of the problem we know the following facts about a circle:

- its center is at the point:

`(h,k)=(1,-2)`

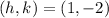
- it's tangent to the y-axis.

Using the data above we draw the following graph of the circle:

From the graph, we see that the circle center is at a distance of 1 unit from the y-axis (to which it is tangent), so the circle has a radius:

`r=1`

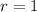
Replacing the data of the center and the radius in the general form equation of the circle, we get:

`\begin{gathered} (x-h)^2+(y-k)^2=r^2 \\ (x-1)^2+(y+2)^2=1 \end{gathered}`