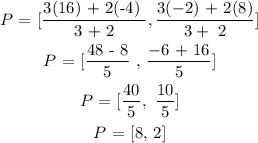Question

Find the coordinates of point P along directed line segment AB with A(-4,8).and B(16,-2), so that the ratio of AP to PB is 3 to 2.

Answer 1

The coordinates of point P along the directed line segment AB with A(-4,8) and B(16,-2) implies that the point P divides the line AB internally

Recall that the formular for the coordinates of a point that divides a line internally is given as

`P\text{ = \lbrack}\frac{mx_2+nx_1}{m\text{ + n}}\text{ , }\frac{my_2+ny_1}{m\text{ + n}}\rbrack`

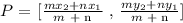
where m and n is the ratio of the internal division

Thus, we have

`\begin{gathered} P\text{ = \lbrack}\frac{3(16)\text{ + 2(-4) }}{3\text{ + 2}},\frac{3(-2)\text{ + 2(8)}}{3+\text{ 2}}\rbrack \\ P\text{ = \lbrack}\frac{48\text{ - 8}}{5}\text{ , }\frac{-6\text{ + 16}}{5}\rbrack \\ P\text{ = \lbrack}(40)/(5),\text{ }(10)/(5)\rbrack \\ P\text{ = \lbrack{}8, 2\rbrack} \end{gathered}`