Question

The fox population in a certain region has an annual growth rate of 9 percent per year. It is estimated that the population in the year 2020 was 24200.(a) Find a function that models the population t years after 2020 ( t=0 for 2020).Your answer is P(t)=(b) Use the function from part (a) to estimate the fox population in the year 2028.Your answer is (the answer should be an integer)

Answer 1

a. The growth of the fox population can be modeled by the following equation:

`P(t)=P_0a^t`

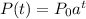
Where P0 is the initial value of the population, a is any number and t is the time in years.

To determine a we have to add 1 and the growth rate in decimal form, it means 0.09:

`\begin{gathered} a=1+0.09 \\ a=1.09 \end{gathered}`

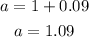
P0 has a value of 24200 which is the fox population in 2020.

The equation that models this situation is:

`P(t)=24200(1.09)^t`

b. To find the fox population in 2028, replace t for 8 (number of years since 2020) and solve:

`\begin{gathered} P(8)=24200(1.09)^8 \\ P(8)=48220 \end{gathered}`

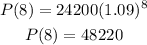
In 2028 there will be approximately 48220 foxes.