Answer:
28.095 cm^2
Step-by-step explanation:
To determine the area of the given shape, we have to determine the area of the complete rectangle, then subtract the areas of each of the cut-out semi-circles as seen below;
From the shape, let's determine the length and width of the rectangle;
Length(l) = 2 cm + 5 cm + 2 cm = 9 cm
Width(w) = 1 cm + 4 cm + 1 cm = 6 cm
So the area of the complete rectangle will be;

Let's now determine the area of the top semi-circle given that the radius is 2cm (radius = diameter/2 = 4/2 = 2cm);
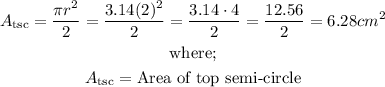
Given that the two semi-circles by the side of the rectangle have the same radius(r) which is 2.5cm (r = diameter/2 = 5/2 = 2.5cm), let's go ahead and determine their area;
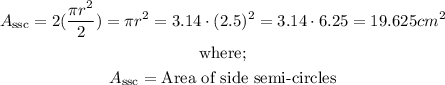
So the area of the shape will be;

Therefore, the area of the shape is 28.095 cm^2