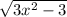Question

The sides of an isosceles triangle are (2x + 1), (2x + 1) and (2x + 4). Treating the longest side as the base, determine an expression for the perpendicular height of the triangle. Give your answer in simplest form.

Answer 1

Drawing a diagram of the triangle we have

Let a be the length of the perpendicular height of the triangle, then we can solve it using the Pythagorean Theorem

`\begin{gathered} a^2+b^2=c^2 \\ a^2+((2x+4)/(2))^2=(2x+1)^2 \\ a^2+(x+2)^2=(2x+1)^2 \\ a^2+x^2+4x+4=4x^2+4x+1 \\ a^2=4x^2+4x+1-x^2-4x-4 \\ a^2=3x^2-3 \\ √(a^2)=√(3x^2-3) \\ \end{gathered}`

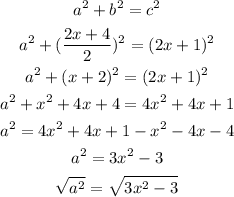
Therefore, the length of the perpendicular height of the triangle can be given in the expression

`√(3x^2-3)`