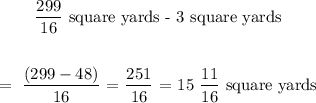Question

Ed wants to hang his posters on a wall that is 3 yards tall and are 5-yards wide. Hehangs 3 posters, each of which is 1 yards high and yards wide. What is the area of the1.2wall that is NOT covered by posters?

Answer 1

The area of the wall not covered by the three posters is 15 11/16 square yards

We are interested in calculating the area of the wall not covered by the 3 posters.

To go about this, we need to know the area of the wall, afterwards, we are going to calculate the area of the wall taken by the posters.

To calculate the area of the wall taken by the posters, we subtract the area of the posters from the area of the wall.

From the question, we can see that the shapes of both the wall and posters are rectangular.

Mathematically, we can calculate the area of the wall as follows;

`\begin{gathered} \text{Area of wall = Length of wall }*\text{ width of wall} \\ \\ =\text{ 3}(1)/(4)\text{ yards }*\text{ 5}(3)/(4)\text{ yards} \\ \\ \text{For convenience sake, we write the fractions in the improper form;} \\ =\text{ }(13)/(4)\text{ }*\text{ }(23)/(4)\text{ = }(299)/(16)\text{ square yards} \end{gathered}`

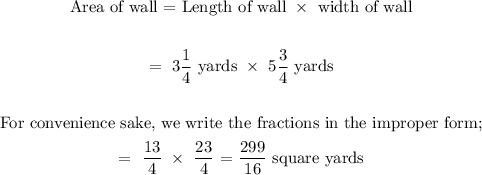
From here, we proceed to calculate the area of the posters;

`\begin{gathered} \text{Area of one poster = Length of poster }*\text{ width of poster} \\ \text{Area of 3 posters = 3( length of poster }*\text{ width of poster)} \\ \\ \text{Area of 3 posters = 3(1}(1)/(2)\text{ }*\text{ }(2)/(3)) \\ \\ \text{Area of 3 posters = 3(}(3)/(2)\text{ }*\text{ }(2)/(3)) \\ \\ \text{Area of 3 posters = 3(1)} \\ \text{Area of 3 posters = 3 square yards} \end{gathered}`

So the area of the wall not covered by the posters will be;

`\begin{gathered} (299)/(16)\text{ square yards - 3 square yards} \\ \\ =\text{ }((299-48))/(16)\text{ = }(251)/(16)\text{ = 15 }(11)/(16)\text{ square yards} \end{gathered}`