Question

In 8 hr, Kerrie can travel 21 mi upriver and come back. The rate of the current is 2 mph. Find the rate of her boat in still water.

Answer 1

`5.92mph`

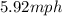
1) We can solve this problem, by considering that we are dealing with ratios. So let's start out writing the equation keeping in mind this relation:

`rate=(d)/(t)`

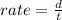
2) So, we can write out this keeping also in mind that Kerrie goes upriver and down the river:

`\begin{gathered} Upriver:r-2mph \\ Downriver:\:r+2 \\ (21)/(r+2)+(21)/(r-2)=8 \\ \\ (21)/(r+2)\left(r+2\right)\left(r-2\right)+(21)/(r-2)\left(r+2\right)\left(r-2\right)=8\left(r+2\right)\left(r-2\right) \\ 21\left(r-2\right)+21\left(r+2\right)=8\left(r+2\right)\left(r-2\right) \\ 42r=8r^2-32 \\ 8r^2-32-42r=42r-42r \\ 8r^2-42r-32=0 \\ r_=(-\left(-42\right)\pm√(\left(-42\right)^2-4\cdot\:8\left(-32\right)))/(2\cdot\:8) \\ r_1=5.925,r_2=-0.67 \\ \end{gathered}`

We can discard negative values for the rate. Then the rate in still water is 5.9 mh