Given the information, we have the following triangle:
then, we can use the formula for the area of the triangle given two sides and the angle between them:
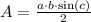
where a, b are the two sides and c is the angle.
In this case, we have the following:

then, using the formula we have the following:
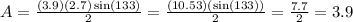
therefore, the area is 3.9cm^2