Answer:
284 square units -----> 1796 cubic units
177 square units -----> 884 cubic units
380 square units -----> 2789 cubic units
95 square units -----> 349 cubic units
Step-by-step explanation:
The area of the largest cross-section of the sphere is equal to
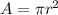
Where r is the radius of the sphere and π = 22/7. Solving for r, we get:
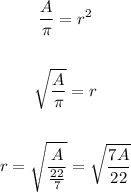
Then, with the radius, we will be able to calculate the volume of the half sphere as follows

Therefore, for each option, we get:


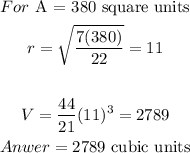
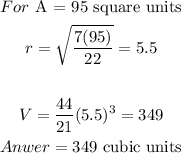
Therefore, the answers are
284 square units -----> 1796 cubic units
177 square units -----> 884 cubic units
380 square units -----> 2789 cubic units
95 square units -----> 349 cubic units