Step 1: Write out the formula
Given the equation of a circle with center (h,k) and radius r, if it is shifted left by a unit and up by b unit, then the function would become
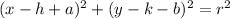
Step 2: Write out the given value and substitute them into the formula
In this case,

Therefore the new equation is given by
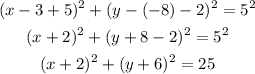
Hence, the new equation for the circle is (x+2)²+(y+6)²=25