24 oz of 12% alcohol and 24oz of 20% alcohol
1) We can solve this problem, writing out a Linear Equation System. So we can write out the following, calling alcohol 12% x, and y an 20% alcohol solution
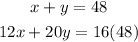
2) So, based on that, the first equation refers to the weight, and the second one to the quantities of each we can write out:
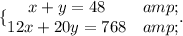
Let's use the Addition/Elimination Method

So we need 24 ounces for the 20% Alcohol solution, let's finally find the quantity for the 12% Alcohol:
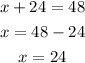
3) Hence, the answer is 24 oz of 12% alcohol and 24oz of 20% alcohol