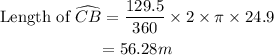Question

In ○ D shown below,€(measure) ADC = €(measure) BDC. Find the indicated measure

Answer 1

• 259 degrees

,

• 112.56m

,

• 56.28m

Step-by-step explanation:

In the circle:

`\text{mArc ADC}\cong\text{mArc BDC}`

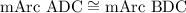
The sum of the angle in a circle = 360 degrees

`\begin{gathered} \widehat{}\widehat{\text{ADC}}\text{+}\widehat{B\text{DC}}\text{+}\widehat{A\text{DB}}=360\degree \\ \widehat{\text{ADC}}\text{+}\widehat{B\text{DC}}\text{+}101\degree=360\degree \\ 2*\widehat{B\text{DC}}\text{+}101\degree=360\degree \\ 2*\widehat{B\text{DC}}=360\degree-101\degree \\ 2*\widehat{B\text{DC}}=259\degree \\ \widehat{B\text{DC}}=(259\degree)/(2) \\ \widehat{\text{ADC}}=\widehat{B\text{DC}}=129.5\degree \end{gathered}`

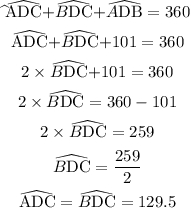
Part 8

The measure of arc ACB.

`\begin{gathered} \text{ }\widehat{ACB}=360\degree-101\degree \\ =259\degree \end{gathered}`

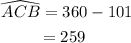
Part 9

Length of arc ACB

`\begin{gathered} \text{Length of an arc=}(\theta)/(360\degree)*2\pi r \\ \theta=\text{central angle} \end{gathered}`

Therefore:

`\begin{gathered} \text{Length of }\widehat{ACB}=(259\degree)/(360\degree)*2*\pi*24.9 \\ =112.56m \end{gathered}`

Part 10

Length of arc CB.

The central angle subtended by CB = 129.5 degrees

Therefore:

`\begin{gathered} \text{Length of }\widehat{CB}=(129.5\degree)/(360\degree)*2*\pi*24.9 \\ =56.28m \end{gathered}`