Question

find the value of the variable. if your answer is not a whole number, leave it in the simplest radical form

Answer 1

The given triangle is a rigth triangle with:

Base= y

Height= x

Hypothenuse =20√3

You have to calculate the value of the variables.

Considering the angle ∠30, the base of the triangle represents the opposite side to the angle and the height represents the adjacent side.

Since we know the measure of the hypothenuse and one angle, we can use the trigonometric functions to determine the values of x and y:

`\begin{gathered} \sin \theta=(opposite)/(hypothenuse) \\ \cos \theta=(adjacent)/(hypothenuse) \\ \tan \theta=(opposite)/(adjacent) \end{gathered}`

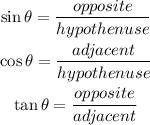
For the variable y, you have to use the sin of 30º

`\begin{gathered} \sin 30=\frac{y}{20\sqrt[]{3}} \\ y=\sin 30\cdot20\sqrt[]{3} \\ y=10\sqrt[]{3} \end{gathered}`

For the variable x, you have to use the cosine of 30º

`\begin{gathered} \cos 30º=\frac{x}{20\sqrt[]{3}} \\ x=\cos 30º\cdot20\sqrt[]{3} \\ x=30 \end{gathered}`

x=30, y=10√3 → the correct option is d.