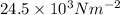The pressure of the reading of tube is given as,
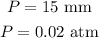
The relation between the before and after pressure is,

where v1 is the velocity of the speed initially, and v2 is the velocity at the final state.
Substituting the known values,
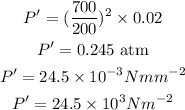
Thus, the final pressure reading of the pilot tube is