Question

The monthly service charge $S of mobile phone is the sum of two parts. One part is a constant and the other varies directly as the connection time t minutes. When the monthly service charge is $230, the connection time is 100 minutes. When the monthly service charge is $290, the connection time is 130 minutes.a) Express S in terms of t.b) Find the value of the connection time t when the monthly service charge$S is $330.

Answer 1

Since the monthly charge S is made from 2 parts,

A constant part, let it b

A part depends on a direct relationship between it and the time t

Then the form of S should be

`S=mt+b`

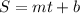
Where:

m is the rate of change

b is the constant amount

Since S = 230 at t = 100

Since S = 290 at t = 130

Substitute them in the equation above to make 2 equations of m, b and solve them

`\begin{gathered} 230=100m+b\rightarrow(1) \\ 290=130m+b\rightarrow(2) \end{gathered}`

Subtract equation(1) from equation (2) to eliminate b

`\begin{gathered} (290-230)=(130m-100m)+(b-b) \\ 60=30m \end{gathered}`

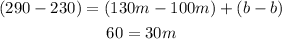
Divide both sides by 30 to find m

`\begin{gathered} (60)/(30)=(30m)/(30) \\ 2=m \\ m=2 \end{gathered}`

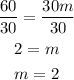
Substitute m in equation (1) by 2 to find b

`\begin{gathered} 230=100(2)+b \\ 230=200+b \end{gathered}`

Subtract both sides by 200

`\begin{gathered} 230-200=200-200+b \\ 30=b \\ b=3 \end{gathered}`

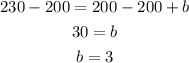
a) The equation of S is (substitute m by 2 and b by 30)

`S=2t+30`

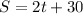
b) Since the monthly fee is $330, then

S = 330

Substitute it in the equation to find t

`330=2t+30`

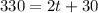
Subtract 30 from both sides

`\begin{gathered} 330-30=2t+30-30 \\ 300=2t \end{gathered}`

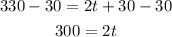
Divide both sides by 2 to find t

`\begin{gathered} (300)/(2)=(2t)/(2) \\ 150=t \\ t=150 \end{gathered}`

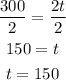
The value of the time is 150 minutes