Question

the area of a rectangle is 135 feet we know that one side is 12 feet longer than three times the other side the rectangle has dimensions a and b where a less than or equal to b so a the shorter side is how many feet

Answer 1

This is our rectangle:

We know that the area of a rectangle is it's length times it's width:

`A=b* a`

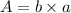
The problem says that one side is 12ft longer than 3 times the other side, so if a is the shorter side:

`b=3a+12`

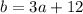
And we know that the area is 135ft². If we replace this and the expression for b into the equation for the area we can clear a and then find b:

`\begin{gathered} 135=b* a \\ 135=(3a+12)a \\ 135=3a^(2)+12a \\ 0=3a^(2)+12a-135 \end{gathered}`

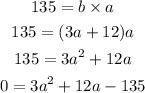
Note that we got a 2 degree equation. We can solve it this way:

`\begin{gathered} 0=Ax^(2)+Bx+C \\ x=\frac{-B\pm\sqrt[]{B^(2)-4AC}}{2A} \end{gathered}`

In our equation we have that A=3, B=12 and C=135 (and instead of x we have a):

`\begin{gathered} a=\frac{-B\pm\sqrt[]{B^2-4AC}}{2A} \\ a=\frac{-12\pm\sqrt[]{12^2+4\cdot3\cdot135}}{2\cdot3}=\frac{-12\pm\sqrt[]{144^{}+1620}}{6}=\frac{-12\pm\sqrt[]{1764}}{6}=(-12\pm42)/(6) \end{gathered}`

One of these results will be negative, but since a is the size of the side of a rectangle we don't want a negative number, so we'll keep the positive result:

`a=(-12+42)/(6)=(30)/(6)=5`

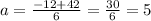
So we got that side a = 5 feet

Then we find b with the expression:

`b=3a+12=3\cdot5+12=15+12=27`

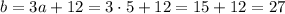
Side b = 27 feet