Let's determine the rate of change of the two functions at 0 ≤ x ≤ 1.
For f(x),
Point 1: 0, -4
Point 2: 1, 2
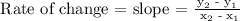
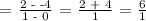
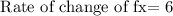
For g(x),
Point 1: 0, 3
Point 2: 1, 9

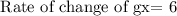
Therefore, both functions has the same average rate of change over this interval.
The answer is CHOICE C.