Given:
object distance (do) = 30 cm
object size (so) = 10 cm
focal length (f) = - 20 cm
Required:
image distance (di),
magnification (m)
image characteristics.
Equations:
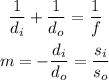
Solution:

Location: 12 cm "inside" the mirror (di = -12 cm)
Orientation: Upright (m > 0)
Size: si = 4 cm (object size multiplied by m)
Type: Virtual image (di < 0)