Question

A medical equipment industry manufactures x-ray machine. The unit cost C (the cost in dollars to make each X-ray machine) depends on the number of machines made. if x machines are made, then the unit cost is given by the function c(x)=0.2x^2-36x+8122. How many machines must be made to minimize the unit cost?Do not round your answer. Number of x-ray machines:

Answer 1

For the minimum value, the first derivative of function is equate to zero and value of second derivative is posiive.

Determine the first derivative of cost function.

`\begin{gathered} (d)/(dx)c(x)=(d)/(dx)(0.2x^2-36x+8122) \\ =0.4x-36 \end{gathered}`

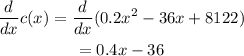
For maximum and minimu value,

`\begin{gathered} 0.4x-36=0 \\ x=(36)/(0.4) \\ =90 \end{gathered}`

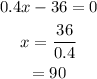
Determie the second derivative of the cost function.

`\begin{gathered} (d^2)/(dx^2)c(x)=(d^2)/(dx^2)(0.2x^2-36x+8122) \\ =0.4 \end{gathered}`

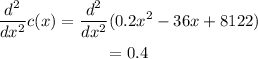
The second derivative of cost function is positive for all value of x. So x = 90 machines corresponds to the minimum value of function.

Answer: 90 machines