Question

I need help with my homework PLEASE CHECK WORK WHEN DONENUMBER 3

Answer 1

The point here is to calculate the area of the curve between 200 to 800 because it's a distribution of probability and even more, it's a normal distribution then look at it we can already see that the standard variation is 100 and the mean is 500, then

Then we must find

`P(200\leq X\leq800)`

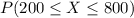
In fact, we are doing

`P(\mu-3\sigma\leq X\leq\mu+3\sigma)`

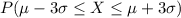
The normal distribution is symmetrical then

`P(\mu-3\sigma\leq X\leq\mu+3\sigma)=2P(\mu-3\sigma\leq X\leq\mu)=2P(\mu\leq X\leq\mu+3\sigma)`

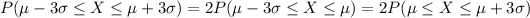
Therefore we can just evaluate

`P(\mu-3\sigma\leq X\leq\mu+3\sigma)=2P(\mu\leq X\leq\mu+3\sigma)`

But why use the standard deviation and the mean? because we already know that value!

`\begin{gathered} P(\mu\leq X\leq\mu+\sigma)=0.3413 \\ \end{gathered}`

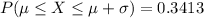
For 2*standard deviation

`P(\mu\leq X\leq\mu+2\sigma)=0.4772`

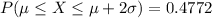
And our case, for 3*standard deviation

`P(\mu\leq X\leq\mu+3\sigma)=0.4987`

Therefore

`\begin{gathered} 2P(\mu\leq X\leq\mu+3\sigma)=2\cdot0.4987 \\ \\ 2P(\mu\leqslant X\leqslant\mu+3\sigma)=0.9974 \end{gathered}`

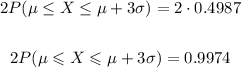
Let's go back to our original equation

`P(200\leq X\leq800)=0.9974`

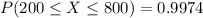
Then 99.74% of the students score between 200 and 800, now just do 99.8% of 1000

`99.8\%\text{ of 1000 = 998 students}`

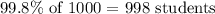
Hence, 998 students scored between 200 and 800